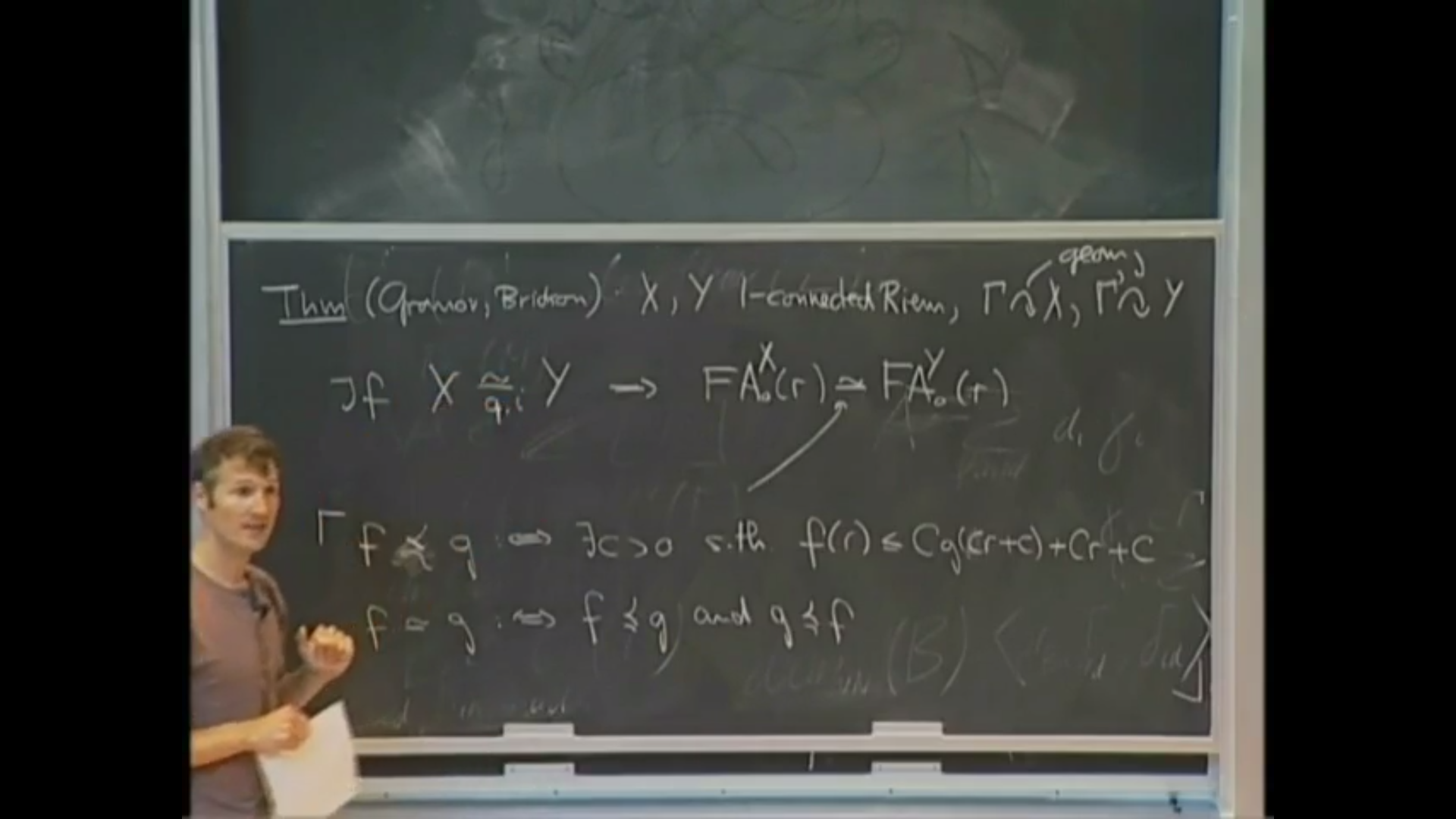Isoperimetric Filling Problems and Large Scale Geometry
Presenter
August 26, 2011
Keywords:
- quantitative geometry
- isoperimetric inequality
- asymptotic geometry
- geometric measure theory
- space-filling curves
- geodesics
- hyperbolic groups
- hyperbolic geometry
MSC:
- 46-xx
- 46Bxx
- 20F67
- 20F65
- 20F69
- 20Fxx
- 20-xx
Abstract
Given a metric space X, the k-th isoperimetric filling function FV_k(r) is the smallest function such that every k-cycle of volume at most r in X bounds a (k+1)-chain in X of volume at most FV_k(r). In this talk, we will explore relationships between the asymptotic growth of FV_k(r) and the large scale geometry of the underlying space X.
Much of the talk will be concerned with the first isoperimetric filling function (i.e. filling curves with surfaces) and we will, in particular, discuss the following two results and indicate how tools from geometric measure theory play a role in their proofs:
(i) if X is a geodesic metric space for which FV_1(r) is bounded above by (1-epsilon)(4\pi)^{-1}r2 epsilon>0 for all sufficiently large r then X must be Gromov hyperbolic;
(ii) there exist simply-connected nilpotent Lie groups of step two for which FV_1(r) does not have the growth of a polynomial function.
The first result is optimal and generalizes results of Gromov, Olshanskii, Bowdich, Drutu, and Papasglu. The second result answers in the negative a long-standing open question in geometric group theory about the possible growth of (isoperimetric) Dehn functions for nilpotent groups.
In the last part of the talk we will discuss results concerning the higher (i.e. k>1) isoperimetric filling functions and, in particular, explain how their growth is related to the Euclidean rank, i.e. the highest dimension of isoperimetrically embedded flats, in spaces of non-positive curvature.